Heterogeneous thin structures with prestrain

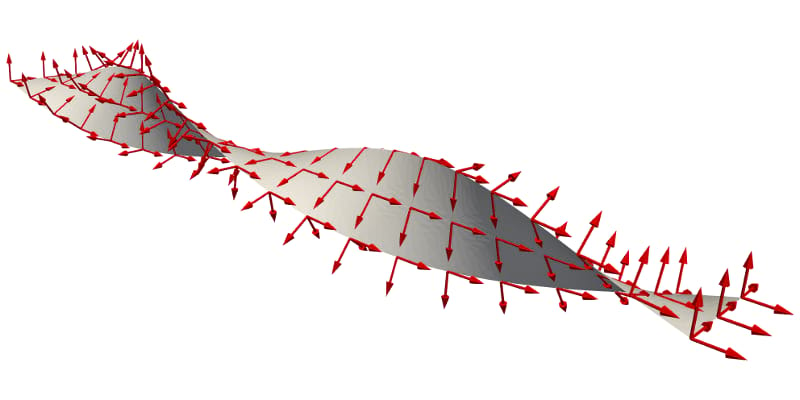
Prestrain and -stress can have a tremendous effect on the mechanical behavior of slender elastic structures leading to, e.g., complex shapes in equilibrium, wrinkling and symmetry breaking. Natural and synthetic materials (including biomembranes as considered in other projects in the research unit) feature residual stress due to growth, swelling, or thermal expansion. These mechanisms are used in the design of active films and rods that undergo a change of shape triggered by an external stimulus (e.g., temperature or humidity). By new fabrication techniques (e.g., additive manufacturing) active composite films and rods can be produced that gain functionality from microstructures.

To design and optimize such devices, effective mathematical models and numerical simulation methods are required. This is challenging since the underlying mechanical models are highly nonlinear and invoke multiple scales. In this project we investigate the influence of prestrain in microstructured thin films and rods with tools from applied analysis and numerical simulation. Starting from a 3D nonlinear elasticity model for prestrained, periodic composites, we will rigorously derive (via Gamma-convergence) homogenized plate and rod models that allow for large bending deformations. They feature an additional spontaneous curvature tensor that captures the macroscopic geometric effects of the microheterogeneous prestrain. We will determine tailor-made cell-problems that allow to effectively compute the large-scale material properties as well as the spontaneous curvature tensor as function of the microstructure. The derived models feature a metric constraint. In the project we develop a reliable geometric finite element method for variational problems that feature such metric constraints. By means of simulations we will investigate the rich connection between the microscopic distribution of prestrain, the composite geometry, and the effective equilibrium shapes of the thin structure.

Project group

• Prof. Dr. Stefan Neukamm
• Prof. Dr. Oliver Sander
• Klaus Böhnlein
• Dr. David Padilla-Garza

Publications

Klaus Böhnlein, Stefan Neukamm, David Padilla-Garza, and Oliver Sander, A homogenized bending theory for prestrained plates, In J. Nonlinear Sci., Vol. 33, pp. 22, 2023. [doi] [bibtex]

Sabine Haberland, Patrick Jaap, Stefan Neukamm, Oliver Sander, and Mario Varga, Representative Volume Element Approximations in Elastoplastic Spring Networks, In Multiscale Modeling & Simulation, Vol. 22, pp. 588–638, 2024. [doi] [bibtex]