Numerical methods for surface fluids
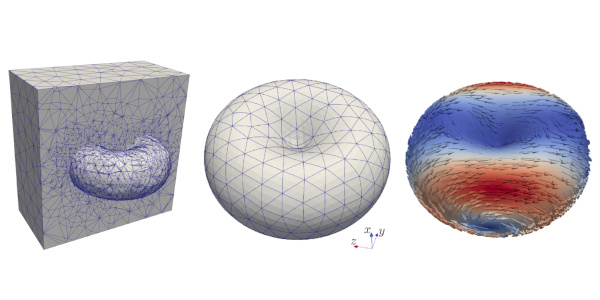
The project considers a class of surface Navier-Stokes equations which are used to model viscous two-dimensional incompressible fluidic surfaces. We restrict to sufficiently smooth closed oriented (evolving) surfaces embedded in . The main topic of the project is the development of efficient and reliable finite element based numerical simulation methods for these surface Navier-Stokes equations. Such methods have been developed for stationary surfaces in the previous funding period and are now extended to evolving surfaces. Besides method development we also define benchmark problems and elaborate rigorous stability and error analyses. Finally, we also develop and investigate model extensions towards two-phase surface flows and active flows.
Publications
| Hauke Sass and Arnold Reusken, An accurate and robust Eulerian finite element method for partial differential equations on evolving surfaces, In Computers & Mathematics with Applications, Vol. 146, pp. 253–270, 2023. [doi] [bibtex] |
| Maxim A. Olshanskii, Arnold Reusken, and Paul Schwering, An Eulerian finite element method for tangential Navier-Stokes equations on evolving surfaces, In Mathematics of Computation, Vol. 93, pp. 2031–2065, 2024. [doi] [bibtex] |
| Arnold Reusken, Analysis of the Taylor-Hood surface finite element method for the surface Stokes equation, In Mathematics of Computation, 2024. [doi] [bibtex] |
| Tilman Aleman and Arnold Reusken, Numerical analysis of a constrained strain energy minimization problem, arXiv e-prints, 2411.19089 [math.NA], 2024. [bibtex] |
| Maxim Olshanskii, Arnold Reusken, and Paul Schwering, A narrow band finite element method for the level set equation, arXiv e-prints, 2407.02950 [math.NA], 2024, accepted for publication in SISC. [bibtex] |
| Arnold Reusken and Hauke Sass, Analysis of a space-time unfitted finite element method for PDEs on evolving surfaces, arXiv e-prints, 2401.01215 [math.NA], 2024. [bibtex] |
| Ingo Nitschke and Axel Voigt, Observer-invariant time derivatives on moving surfaces, In Journal of Geometry and Physics, Vol. 173, pp. 104428, 2022. [doi] [bibtex] |
| Veit Krause and Axel Voigt, A numerical approach for fluid deformable surfaces with conserved enclosed volume, In Journal of Computational Physics, Vol. 486, pp. 112097, 2023. [doi] [bibtex] |
| Elena Bachini, Veit Krause, and Axel Voigt, The interplay of geometry and coarsening in multicomponent lipid vesicles under the influence of hydrodynamics, In Physics of Fluids, Vol. 35 (4), pp. 042102, 2023. [doi] [bibtex] |
| Michael Nestler and Axel Voigt, A diffuse interface approach for vector-valued PDEs on surfaces, arXiv e-prints, 2303.07135 [math.NA], 2023. [bibtex] |
| Ingo Nitschke and Axel Voigt, Active nematodynamics on deformable surfaces, arXiv e-prints, 2405.13683 [cond-mat.soft], 2024. [bibtex] |
| Veit Krause and Axel Voigt, Wrinkling of fluid deformable surfaces, In Journal of the Royal Society Interface, Vol. 21 (216), 2024. [doi] [bibtex] |
| Maik Porrmann and Axel Voigt, Shape evolution of fluid deformable surfaces under active geometric forces, In Physics of Fluids, Vol. 36 (10), 2024. [doi] [bibtex] |
| Ingo Nitschke and Axel Voigt, Beris-Edwards Models on Evolving Surfaces: A Lagrange-D'Alembert Approach, arXiv e-prints, 2311.06240 [math-ph], 2023. [bibtex] |
| Elena Bachini, Veit Krause, Ingo Nitschke, and Axel Voigt, Derivation and simulation of a two-phase fluid deformable surface model, In Journal of Fluid Mechanics, Vol. 977, pp. A41, 2023. [doi] [bibtex] |






