Rate-independent evolution of prestrained plates
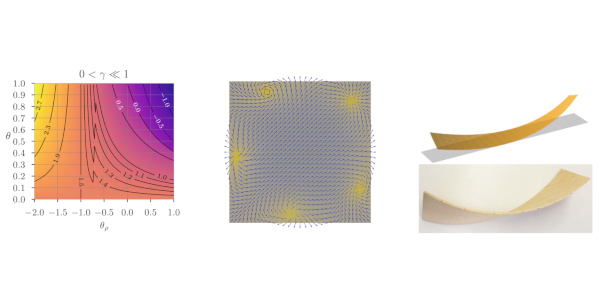
Prestrain has a huge impact on the mechanical behavior of thin elastic plates and films. It can be the result of different physical mechanisms and can be triggered by different stimuli-a property that makes such materials interesting for the fabrication of functional materials with a controlled shape change. Scales range from polymer films of sizes in the (sub-)millimeter range to large composite structures of several meters. An example for the latter are self-shaping wood bilayers, which are currently becoming relevant for complex, free-form timber architecture. In the previous funding period we studied elastic composite plates with a microheterogeneous prestrain. From three-dimensional nonlinear elasticity, we rigorously derived a homogenized bending plate model with an effective prestrain that triggers spontaneous bending. The derived model predicts non-flat equilibrium shapes that depend on the specification of the three-dimensional composite in a complex way. We explored this “microstructure-shape relation” by combining numerical and analytical methods. So far, the prestrain has been a fixed, prescribed quantity. In the second funding period we will consider prestrain that is governed by a field variable that obeys an additional constitutive law. We shall consider variational, static equilibrium problems as well as models that involve a rate-independent evolution and a multiplicative decomposition of the deformation gradient. We will focus on two representative models, namely a swelling model (with the drying process of self-forming wood bilayers in mind) and finite elasto-plasticity. We plan to rigorously derive effective, static and time-dependent plate models with reduced complexity via (evolutionary) Gamma-convergence from three-dimensional models and we shall develop reliable numerical methods to simulate the reduced models. Furthermore, we plan to explore the mechanical behavior of the models combining analytical approaches and numerical simulations.
Publications
| Klaus Böhnlein, Stefan Neukamm, Markus Rüggeberg, and Oliver Sander, Dimension-reduced mathematical modeling of self-shaping wooden composite bilayers, In Wood Material Science & Engineering, pp. 1–11, 2024. [doi] [bibtex] |
| Stefan Neukamm and Kai Richter, Dimension reduction for elastoplastic rods in the bending regime, arXiv e-prints, 2409.08646 [math.AP], 2024. [bibtex] |
| Sabine Haberland, Patrick Jaap, Stefan Neukamm, Oliver Sander, and Mario Varga, Representative Volume Element Approximations in Elastoplastic Spring Networks, In Multiscale Modeling & Simulation, Vol. 22, pp. 588–638, 2024. [doi] [bibtex] |






